When we talk about spacetime, we are describing the three dimensions of space and a fourth dimension that is perpendicular to all three spacial dimensions, which we call time. This implies that space and time are different dimensions of the same thing. This framework is referred to as “3 + 1 spacetime”.
Perpendicularity
We can’t imagine a spacial dimension that is perpendicular to a 3-D coordinate system, as there appears to be no more right angles possible.
To imagine what could be perpendicular to 3-D space, lets redefine how to compose higher dimensions using lower ones.
Ask yourself the question: what is perpendicular to a 0 dimensional point?
Separation
With no dimensional constraints at the 0th dimension, the description of perpendicular doesn’t really cut it. There is no right angle to a point. The way forward is to think of something that is entirely separate. This is the real condition that defines the dimensions.
Let’s place another 0-D point at a location where it can never interact with the previous, and fill the space between and extending outward from with an infinite amount of points, and then you have a 1-D line, the cumulative sum of this process. An infinite amount of separation.

Now consider to go from 1-D to 2-D, you repeat the same process. There is only one line that is entirely separate from the first, that line being parallel. fill the space between and extending, and you get a continuous 2-D plane.
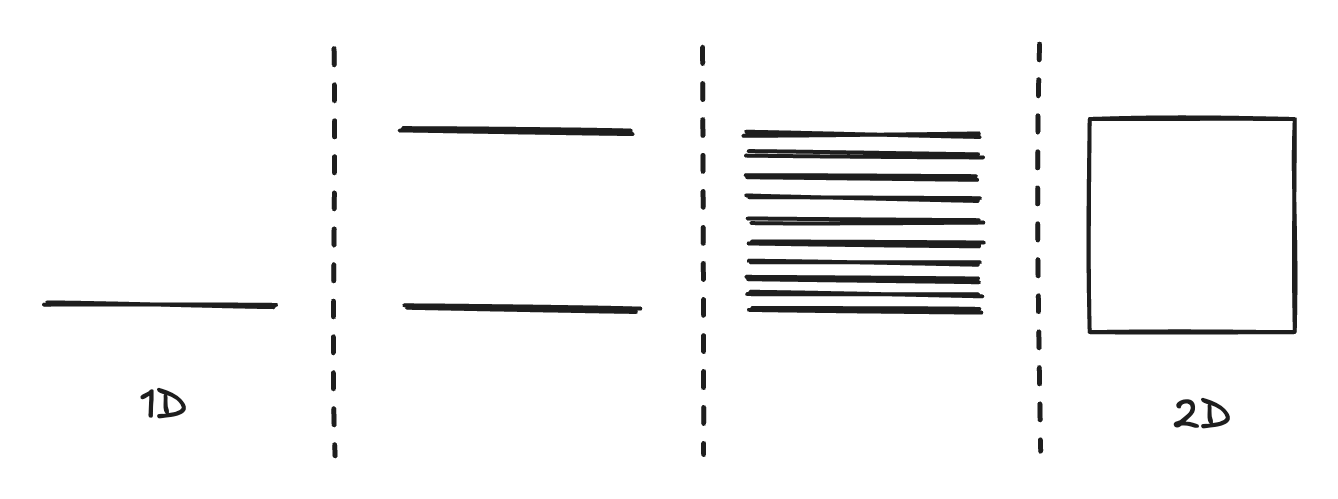 And again with a parallel plane up to 3-D.
And again with a parallel plane up to 3-D.
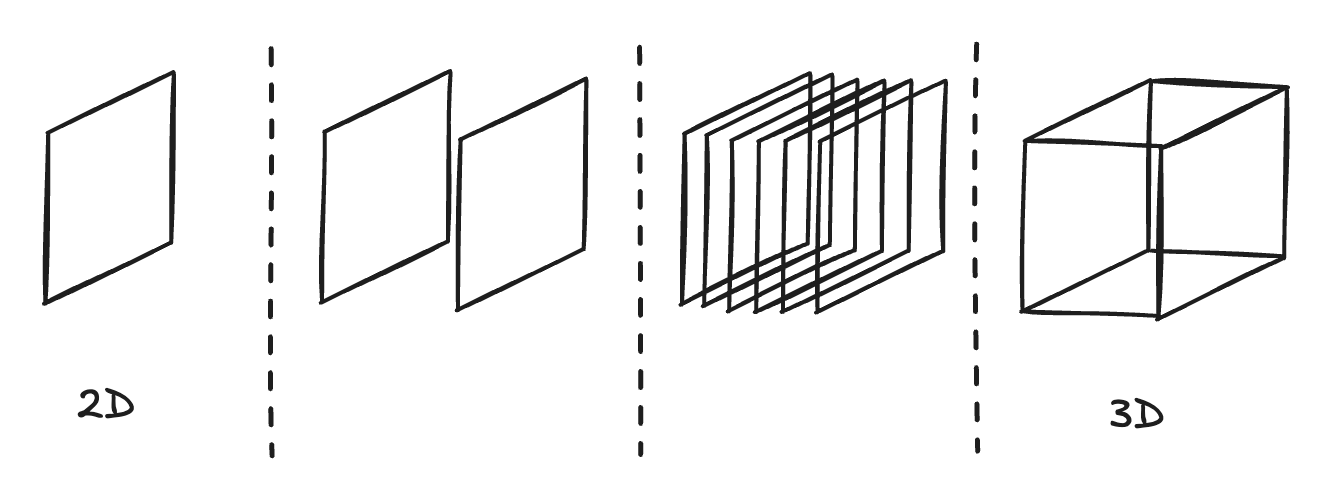
Instead of thinking about 4-D as being perpendicular to 3-D, lets try to find a way to make two 3-D spaces that are entirely separate.
Change
Lets confine an infinite 3 dimensional grid as a box. This box is the third dimension. It includes the entire spacial universe, at one moment in time. It is space.
Now take another one of these boxes, and place it beside the first. This can be thought of as entirely separate from the first box. It is the entire spacial universe, in a specific state.
Now add another box, and another, and another, until you have a long ‘line’ of boxes. This line represents the temporal dimension of space time, or in other words, expresses different states of the spacial dimensions, which when ordered in a sequence, we refer to as change.
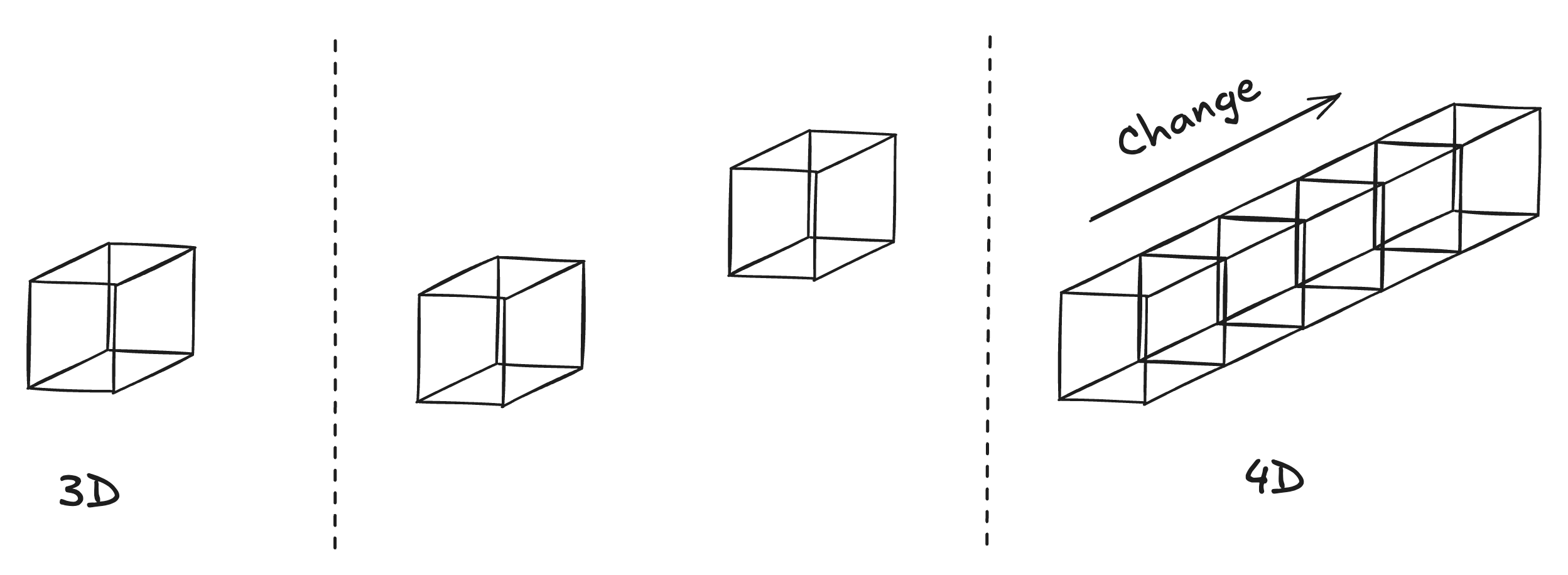
This line is called a time-line.
Imagine yourself zooming out. Watch the box get smaller in your mind, until eventually it becomes so small that is appears to just be a point, quite indistinguishable from the 0-D point from earlier.
The 4-D timeline was created in the same way as a 1-D line, except we are 3 dimensions higher.
The entire 3-D spacial universe behaves similarly to a 0-D spacial point when factoring time. It is space at a point in time. We can now refer to 3-D as a ‘time-point’, and 4-D as a ‘time-line’.
Might this imply that 4-D might behave similarly to 1-D as well?
When you hold a roll of film in your hand, you hold the entire movie at once. The beginning, middle, and end. The film is unchangeable, different events won’t happen on a rewatch. It can only happen one way. The fourth dimension is a bit like film.
When the film plays, it has a certain frame rate. We also experience a flow of time. Read: Falling through time.
This isn’t the end of the story. We can imagine things happening differently. A different ending to your favorite film. There are many edits of it in production where different events did happen.
Continue to The 6 dimensions.